
The Astrophysics Spectator
The Sun will one day have a radius comparable to Earth's. Once it has burned its core hydrogen and helium, it will shrink until it is stabilized by electron degeneracy pressure, becoming a degenerate dwarf (white dwarf) composed principally of carbon.
It may seem odd that something as massive as the Sun should become much smaller than Saturn, but this is the nature of degeneracy pressure. Unlike the pressure exerted by atoms, which changes dramatically with small changes in density, degeneracy pressure changes moderately with density. The consequence is that while the radius of an object supported by the pressure exerted by atoms rises with increasing mass, the radius of an object supported by degeneracy pressure falls with increasing mass, and the most massive degenerate objects are the smallest.
The maximum mass of a degenerate object is set by instability to gravitational collapse. A degenerate object is unstable when the Fermi energy of the degenerate particles providing the pressure exceeds the rest mass energy of one of these particles. This simple condition makes the minimum size of a neutron star or a degenerate dwarf easy to estimate. The radius of a pressure-stabilized body is approximated by equating the gravitational potential energy of a particle providing the mass equal to the temperature within the body, which is the Fermi energy in a cold degenerate object. Setting the Fermi energy to the rest-mass energy of the degenerate particle gives the following relationship:
G M mg/R ? mdc2
In this equation, G is the gravitational constant, c is the speed of light, M is the mass of the object, R is the radius of the object, mg is the mass of the particle providing the gravitational field, and md is the mass of the particle providing the degeneracy pressure. This means that the radius of a degenerate object at the mass of instability is approximately
R ? (G M/c2) ( mg/md)
The radius is therefore of order the Schwarzschild radius (the Schwarzschild radius, which is the radius of a black hole's event horizon, is twice the value in the first set of brackets) times the ratio of the gravitating particle's mass to the degenerate particle's mass.
In a neutron star, protons and neutrons generate both the gravitational field and the internal pressure, so the gravitational potential energy of a proton is about equal to the rest mass energy of the proton. This makes the escape velocity at the surface of a neutron star close to the speed of light, giving the neutron star a size comparable to that of a black hole of the same mass. More precisely, neutron stars are of order 15 km in radius, while a 2 solar-mass black hole has a Schwarzschild radius of 6 km and a last-stable-orbit radius of 9 km. Therefore, the effects of general relativity are strong at the surface of a neutron star. This affects both the appearance of the neutron star and the precise mass at which a neutron star becomes unstable. The dominance of general relativity within a neutron star is a direct consequence of the particles providing the mass also providing the degeneracy pressure.
Within a degenerate dwarf at the Chandrasekhar limit, electrons provide the degeneracy pressure while protons and neutrons provide the mass. This division of roles causes the degenerate dwarf to be larger than a neutron star at its maximum mass limit by about a factor of 2,000, which is the ratio of the proton mass to the electron mass. For this reason, the degenerate dwarf is of order the size of Earth.
The Chandrasekhar limit is itself directly set by fundamental constants of physics, so the sizes of both the neutron star and the degenerate dwarf are also set by the fundamental constants of physics. In terms of fundamental constants of physics alone (numerical factors are ignored), the Chandrasekhar limit is of order M ? (hc/G)3/2mp?2, where h is the Planck constant and mp is the mass of the proton. Because the upper limit on the mass of the neutron star is of order the Chandrasekhar limit, the radius of a neutron star is of order R ? G1/2(h/c)3/2mp?2. The radius of a degenerate dwarf is then approximately R ? G1/2(h/c)3/2mp?1 me?1, where me is the mass of the electron. This shows that the radii of both the neutron star and the degenerate dwarf are fundamentally set by the mass of the proton, with the radius decreasing as the proton mass rises.
Neutron stars have a narrow mass range; they are sandwiched between the Chandrasekhar limit of 1.4 solar masses at the lower end and an instability mass of 2.5 to 5 solar masses at the upper end?the upper limit is set by the rest mass of the proton, the effects of general relativity, and the interactions among the particles within the neutron star, with the last effect accounting for the uncertain. The degenerate dwarf, on the other hand, has a much broader range of radii, because it has a mass that is bounded by the mass of thermonuclear fusion?0.07 solar masses?and the Chandrasekhar limit of 1.4 solar masses. The class of electron-degenerate objects, of which the degenerate dwarfs are the heaviest members, has a lower mass limit at the mass of Saturn, or about 310?4 solar masses. With such a broad range of masses, the electron-degenerate objects have a broad range of radii.
How the radius of an electron-degenerate object changes with mass is found by equating the gravitational potential energy of a proton within the object to the Fermi energy of the electrons. The Fermi energy is set by the electron density. In quantum mechanics, the number of electron energy states below a particular value of kinetic energy is proportional to the energy to the 3/2 power. This means that in cold degenerate matter, where the electrons occupy the lowest energy states, the density of electrons is proportional to the Fermi energy to the 3/2 power. Because the electron density is proportional to M/R3, the Fermi energy is proportional to (M/R3)2/3. The gravitational potential energy of a proton, on the other hand, is proportional to M/R. Equating these two relationships shows that the radius is proportional to M?1/3. Because electron-degenerate objects span a factor of 4,7000 in mass, the least massive electron-degenerate object has 17 time the radius of the most massive object under this simple relationship.
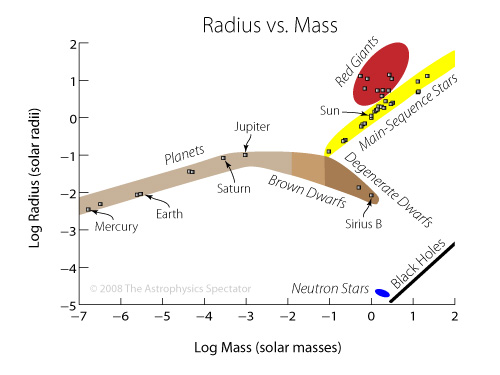
Radius versus mass of self-supporting objects. The giant gaseous planets, brown dwarfs, and degenerate dwarfs, which span about 4 decades along the brown band in the figure, are all supported by degeneracy pressure. The figure is described in detail on the page describing the sizes of objects.
This relationship between mass and radius is displayed in the diagram given above. How the radius of the electron-degenerate object changes with mass can be seen in the radius of Saturn relative to that of the degenerate dwarf Sirius B. Sirius B is 1 solar mass, but it has a measured radius of only 0.0084 solar radii. Saturn, on the other hand, has a mass of 310?4 and a radius of 0.083 solar radii. Sirius B is 3,000 times as massive as Saturn, but 0.1 times the radius. A straight application of the simple M?1/3 relation for the radius of a cold degenerate object give a radius for Sirius B that is 0.07 times the radius of Saturn, which is rather close to the actual value considering that we have ignored differences in composition?hydrogen and helium versus carbon?and other complications in the physics. Simple electron degeneracy explains the basic features of the electron degenerate objects.
One complication to the simple picture outlined above is presented by Jupiter. Unlike Saturn, Jupiter is not yet fully degenerate, because its internal temperature is above the Fermi energy. This high temperature makes Jupiter larger than it would be if completely cold. The presence of internal heat is expected to make most observed giant gaseous planets and brown dwarfs larger than their zero-temperature radii. In fact, the heaviest observed brown dwarfs appear to have radii that are comparable to Jupiter's, despite having masses that are 13 times Jupiter's mass, because they are still warm inside. When they reach zero temperature in the distant future, they should have radii that are half of Jupiter's zero-temperature radius.
